AUTHOR
Natasha Singh
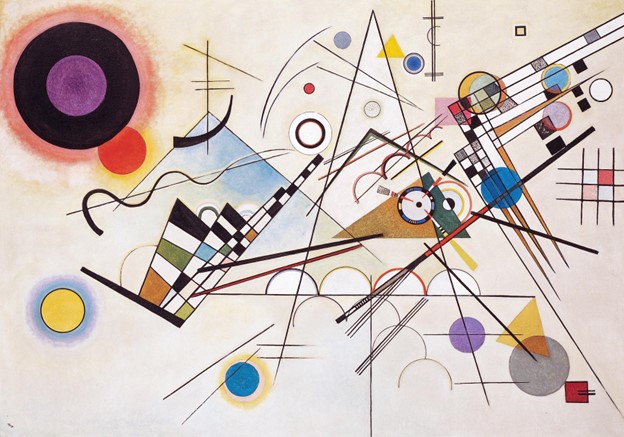
Trains, flyovers, or roads—they all stir a dream of a musical skyline. The patterns and shapes of the old buildings and minarets, when met from a moving vehicle, create an orchestra in the theatre of my mind. Walking through an urban landscape, then, is like an “alert reverie, allowing the fiction of an underlying pattern to assert itself.”i The city skyline, emerging out of various shapes and forms, “transposes [itself] onto the plane of mind” ii, making me drift to a land where I can create an incessant “stream of second attention awareness” iii. The New York skyline looks geometrical, replete with straight lines and right angles, whereas the skyline of Istanbul exhibits an abundance of symmetrical recursive patterns. These repetitive structures feel musical to me and make me wonder how different geometrical forms surrounding us would sound. Will the skyline of New York be different from Istanbul’s skyline sonically?
Unique structures come together to capture the essence of a city. Here, I want to investigate the ways in which spherical, dome-like shapes present a contrast to the linearity of a rectangle, sonically. How does the formation of a shape influence the sound it generates? Structural dimensions, though measurable, have an intrinsically musical character—this draws upon Kandinsky’s argument in Point and Line to Plane that elements are “sounds”: “Form itself, even if completely abstract…has its own inner sound.”iv He deduced, therefore, that all art-forms (dance, architecture, sculpture, poetry) have an elemental sound.
What could be a way to bring out the “inner sounds” from the shapes we see all around us? Can we bring out a common grammar for this correlation? Can the parameters of sound and line be interlinked? What would shapes sound like if we listened to them at an elemental level? Through this essay, I am considering the relationship between a point and sound, treating them as “isomorphic” v, which then enables us to map one’s properties onto the other’s, i.e., the geometry of a shape like its X and Y parameters or angular changes, onto sonic properties such as notes (pitch), velocity, duration.

At the elemental level, we must imagine a point traversing in “spaceland”vIi: the higher it goes, the higher the pitch it produces, the lower it goes, the deeper the bass sound. As Edwin Abott states in his book Flatland, space is a “thoughtland.” viii Space, in which points make a line and lines make shapes. These elements of geometry make an architecture in my mind which has a language to it, an internal sound—Kandinsky argues that “the geometric Point belongs to language and signifies Silence.” ix
Imagine your index finger tracing a point as it moves. A stationary, silent point is given a force which makes it dynamic, and this change of state leads to the formation of a line. Kandinsky describes movement as having two elements: tension and direction. He writes, “tension is the force living within the element”x and then there is direction. A line produced with tension from a point has a sense of direction–up and down or sideways, strictly on an XY plane. The direction of a line dictates its musical nature.
Just as sound is a function of time, the length of a line “is a concept of time.”xi Just as we hear the tonal features of a sound, we can perceive the tonal features of a line– its length, the thickness with which its drawn, the direction it takes.
Let’s illustrate this:
A point moving across lines
In the above experiment, we could notice the frequency of sound being altered by the shape of the line. Another thing to notice was that the repetition of the line gave a rhythm to the sounds we heard. Regular repetitions in a line allow for the creation of a metronome-like rhythm, generating a sound with regular intervals. It is safe to say that our experiment works in accordance with Kandinsky’s statement: “Repetition heightens the inner vibration and source of elementary rhythm.”xii All forms then have a ”primitive rhythm”xiii—it is the measure of “proto-sound”xiv moving repeatedly, over the XY plane, that gives a shape a rhythm, a metre. This is the “spatialisation of musical time”xv. The “proto-sound” moves through the line repeatedly at regular intervals, producing the same sound as when it traces the repetitive nature of the line, which Lefebvre calls the “linear rhythm”xvi of a metronome.
In these experiments, I am trying to explore the rhythmic quality of a shape, by defining some rule sets for how the “proto-sound” absorbs the quality of a line or a shape.
A point moving across a triangle
A point moving across a square
A point moving across a pentagon
Here the thing to note is that geometrical points in a line act as placements for musical notes. I am playing the marimba, a musical instrument, for all the shapes now, for you to focus on the experiment. With the same notes (C4) being triggered at the vector points of the shape, what we hear is a “linear rhythm”, like a metronome.
Can we break away from the repeated tick-tock sound? What If we map the notes from C4 to F5?
Sonically mapping a triangle
Sonically mapping a square
Sonically mapping a pentagon
We can hear a difference in the notes and its progression. Mapping notes from C4 to F5 onto vector points on the Y axis, we arrive at the melodies of elementary shapes like a square or a triangle. These shapes preserve their internal melody by effectuating their geometry on an XY plane, which in turn acts like a ringtone for the shapes.
Let’s run another experiment where we change a regular shape to something irregular. Does this change bring in rhythm too?
A point moving across a square with a difference (notes are not mapped)
A point moving across a square with a difference (mapping sound on the Y axis)
A point moving across a triangle with a difference (notes are not mapped)
A point moving across a triangle with a difference (mapping of sound to y axis)
While a linear progression of a “proto-sound” represents quantified, measured time, “rhythm brings with it a differentiated time, a qualified duration.”xvii. An alteration to a repeated pattern whereby notes playing at a regular interval become altered, which is to say that if we change the length of the notes by altering the shape, the “difference” in the musicality of a shape brings with it the “definition and quality of a rhythm.”xviii
What happens when two or more shapes are played together? Does it add harmony to the sound we hear?
Two shapes playing together
Here, for example, the square acts as an “external measure” just like a metronome, or like a clock’s progression. Whereas, the altered shape of a circle brings in a change in the regular state. It can dilate or constrict: the note lengths vary and, in this case, there are longer silences than expected, thus producing an “internal measure”. That’s the idea of synchronicity between two shapes. This “double measure”, Lefebvre explains, “superimposes” its components that nevertheless “cannot be conflated.”xix
With the convergence of these ideas and experiments, I want to conclude the essay with the questions I had posed in the beginning. Can we find rhythm in the shapes and structures of the city skyline? The highs and the lows of the repeated pattern which mark the signature of the cityscape, can they also produce a distinct soundscape? Applying the same principles as discussed above, we ran the Sound and Structure experiment on two different styles of architecture, one of New York and the other of Istanbul.
New York skyline
Istanbul skyline
The city skyline has its own tonality, not to mention choice of instruments. The symmetries and repetitive forms in the Hagia Sophia of the Istanbul skyline function as an overarching context to drive the tone, whereas the New York cityscape has a distinctive rhythm of contrasting tones. The sounds I now hear when I see shapes, regular or irregular, have assumed new meanings in light of these forays. For these explorations, I would like to credit Mike CJ, a creative technologist I worked with on various fun experiments.
NOTES
i. Sinclair, Iain. Lights Out for the Territory. London: Penguin UK, 2003. Chapter 1
ii. Ibid., Chapter 2
iii. O’Rourke, Karen. Walking and Mapping, Artists as Cartographers. Cambridge: The MIT Press. 2013. 7.
iv. Kandinsky, Wassily. Point and Line to Plane (Dover Fine Art, History of Art). Dover Publications. 1980. 83.
v. Hofstadter, Douglas. Godel, Escher, Bach: An Eternal Golden Braid. New York: Basic Books. 1999. 49.
vi. Kandinsky, Wassily. Point and Line to Plane (Dover Fine Art, History of Art). Dover Publications. 1980. 43.
vii. Abbott, Edwin A. Flatland: A Romance of Many Dimensions. Ingram Short Title. 2018. 124.
viii. Ibid.
ix. Kandinsky, Wassily. Point and Line to Plane (Dover Fine Art, History of Art). Dover Publications. 1980. 25. 101.
x. Ibid., 57.
xi. Ibid., 65.
xii. Ibid., 38.
xiii. Ibid., 95
xiv. Ibid., 65.
xv. Lefebvre, Henri. Rhythmanalysis, Space, Time and Everyday Life. London: Bloomsbury. 2021. 70.
xvi. Ibid., 97.
xvii. Ibid., 86.
xviii. Ibid., 87.
xix. Ibid.
ABOUT THE AUTHOR
Natasha Singh likes to see patterns unfold with the interplay of Indian art and creative coding. She is an interdisciplinary artist, producing sculptures from movement-based art forms and kinetic installations from traditional drawings. As part of the research and development lab at TimeBlur, an award-winning Digital Experience studio, she explores the scope of disruptive technology to digitally conserve India’s arts and culture. Her work has been featured in The Hindu, Bangalore Mirror, Vice, The Creators Project and on Discovery Channel. Techniques like image processing, surface-filling curves, and generative art are applied in most of her current projects. Her work has been showcased at Codame San Francisco, IIT Powai Techfest, IISc Bangalore Innofest, Kala Ghoda festival, ZKM Max Mueller, Magnetic Field Festival Rajasthan, Mini-Maker Faire Bangalore. Natasha is a two-time TEDx speaker and hosts annual community workshops in making art with creative technologies.
To see all the content we have to offer, login below
OR
Don't have an account?
REGISTER FOR FREE
Registration is completely free, stay connected to Serendipity Arts